I awoke early this Christmas morning to watch the successful launch of the James Webb Space Telescope. I remember the genesis of the telescope a quarter of a century ago when it was called the Next Generation Space Telescope. (The telescope’s name is controversial, and I would have preferred an astronomer’s name.) Its development has been prolonged and difficult, as the graphs below attest, but like its predecessor, the Hubble Space Telescope, I am confident of its revolutionary importance — if the upcoming weeks of deployment and months of commissioning succeed.

Development of the unprecedented Webb telescope has been long and challenging.
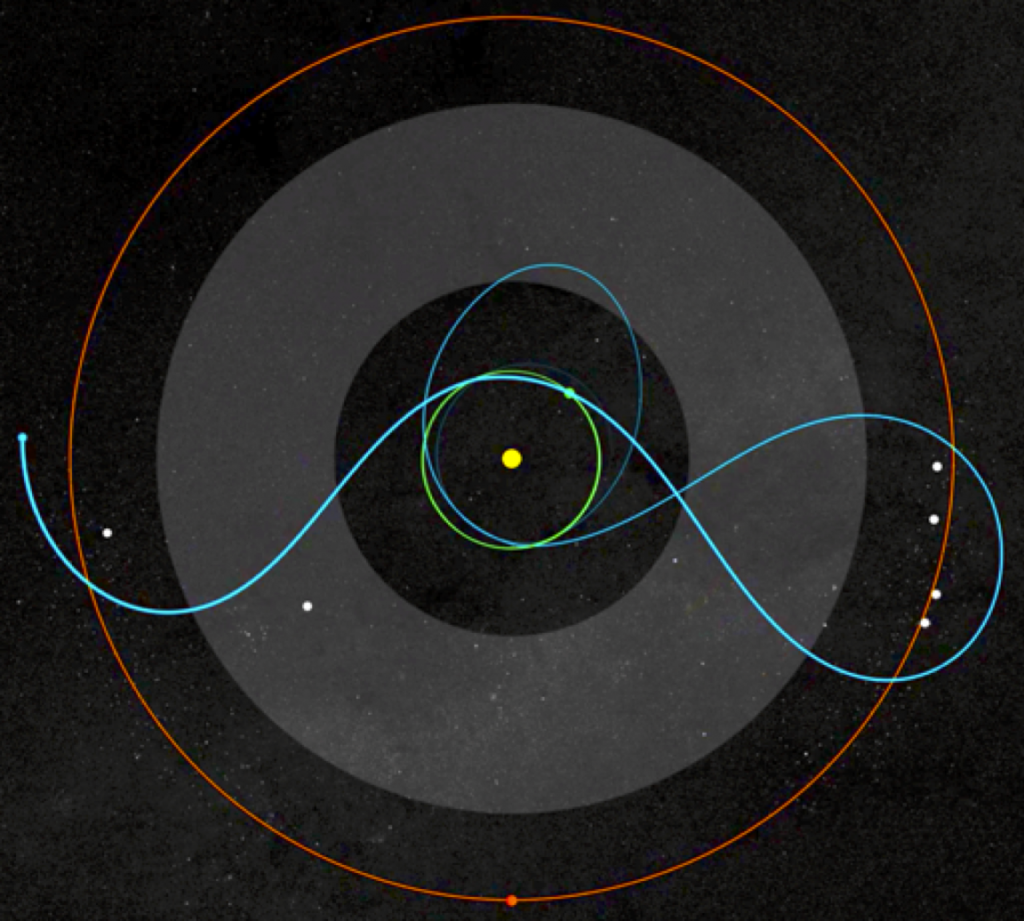
Hubble is a warm visible light telescope, with some near infrared and ultraviolet capability, in a low 540-kilometer Earth orbit. Webb will be a cold infrared telescope, with some red and orange capability, in a halo orbit about the second Earth-Sun Lagrange point 1.5 million kilometers anti-sunward from Earth.
(L_2 is an unstable equilibrium point: in an inertial reference frame, solar and terrestrial gravity combine to pull a mass into a solar orbit with a larger radius but the same period as Earth’s, so the mass appears to hover almost a million miles above Earth’s center; in a frame rotating with Earth about Sun, the combined gravitational force inward balances the centrifugal pseudo-force outward.)
Webb is optimized to study the early universe. Light from the first galaxies is both fainter due to distance and redder due to the expansion of the universe, so a larger, colder, more remote telescope is desirable. By contrast, Hubble is warm enough that its own infrared emission blinds it to this light.
During Webb’s long gestation, exoplanet science dramatically bloomed. Exoplanets are easier to detect in infrared light where the contrast between the glow of a star and its exoplanets is less. Consequently, the study of exoplanets and their atmosphere will be another Webb focus.
First though, Webb must survive six months of terror as it unfolds its giant sunshield and segmented mirror through hundreds of single points of failure.





Thanks, Mark! I enjoy reading your posts as well.