I’ve been fascinated by aperiodic tilings of the plane since Martin Gardner first wrote about them in Scientific American. In the 1960s, Robert Berger discovered a set of 20 426 prototiles or tile-types that can tile the plane but only with no translational periodicity — a wonderful mix of the expected and the surprising, a kind of visual music.
Over the years, the number of required prototiles has been greatly reduced. In the 1970s, Roger Penrose discovered a set of just two concave aperiodic prototiles. Robert Ammann then dissected these to discover a set of three convex aperiodic prototiles. Can a single prototile, one tile or stone, literally ein stein in German, force a nonperiodic tiling? Despite several near misses and potential applications to quasicrystals, the existence of an ein Stein remains a fascinating unsolved problem.
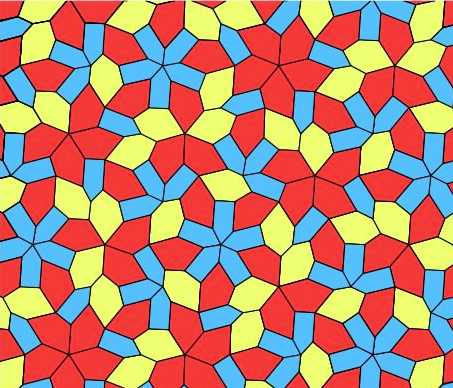
Three convex Ammann tiles force a nonperiodic tiling of the plane.

Two concave Penrose tiles force a nonperiodic tiling of the plane.

3 responses to “Ein Stein”
Excellent examples – thanks.
Still remains a fascinating but not unsolved problem anymore
David Smith’s ein stein is an awesome shape!