Spring is a big time for outreach here at Wooster Physics. The Physics Club runs demonstrations for local elementary schools, doing often two outreach visits a week during the spring. (In the fall, we are usually prepping for this flurry of events — sending letters to the schools and doing scheduling, and training new students on the outreach activities.)
We also have two big events on campus. The Physics Club runs Science Day, an event for all science clubs on campus to do demos and fun activities for the whole community. And we participate in Expanding Your Horizons, a huge event specifically for middle school girls that incorporates not just women science students and professors from the campus but also professional women from around the community whose job includes an aspect of science.
At Science Day, it’s fun to see what the other sciences on campus are doing. The neuroscience club gets a lot of interest with the brains that they bring.



New this year was a giant size demo from the Astronomy Club to demonstrate how massive objects warp the spacetime around them so that other smaller objects orbit the massive one. This was lots of fun to play with!

Air pressure is always a favorite, of course, with the liquid nitrogen parts. This year the demo even attracted President Bolton! I think she had a fun morning with lots of physics — it’s probably a good change from administration.



For Expanding Your Horizons, I do the same workshop three times for different groups of girls. We do the “Humpty Dumpty” experiment, where the girls have about 20 minutes with limited materials to create a container to try to protect an egg from breaking during a fall. We drop the eggs from the 3rd floor, so it’s pretty challenging! This year, Dr. DeGroot joined me and we had lots of fun. I love seeing the creativity of the girls — not only in making their containers, but also in decorating and naming their eggs.




And the moment of truth — dropping the eggs from a great height! If the eggs survive, we add them to the Egg Hall of Fame!
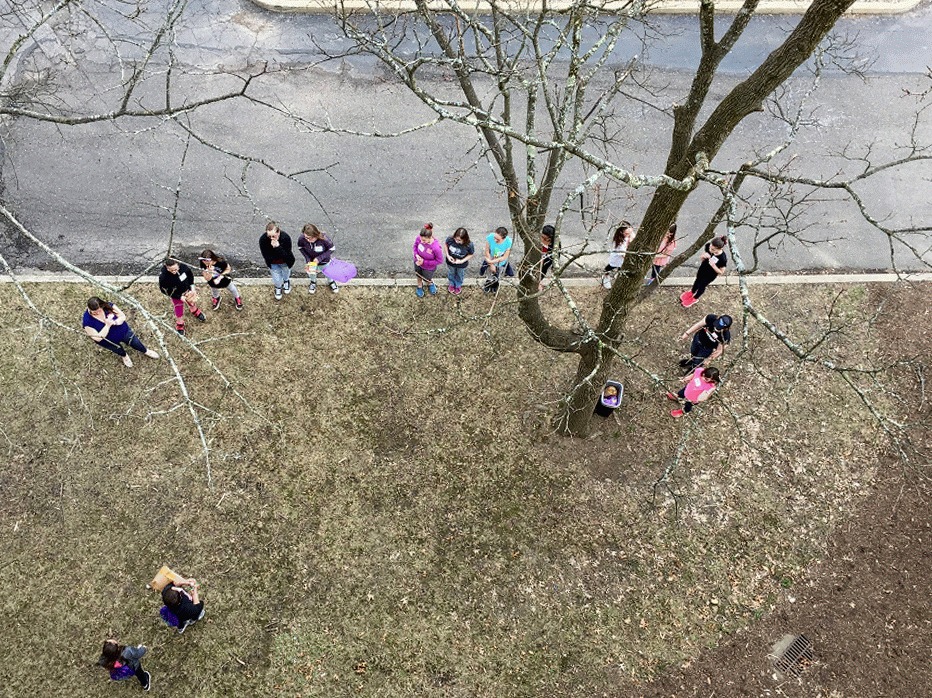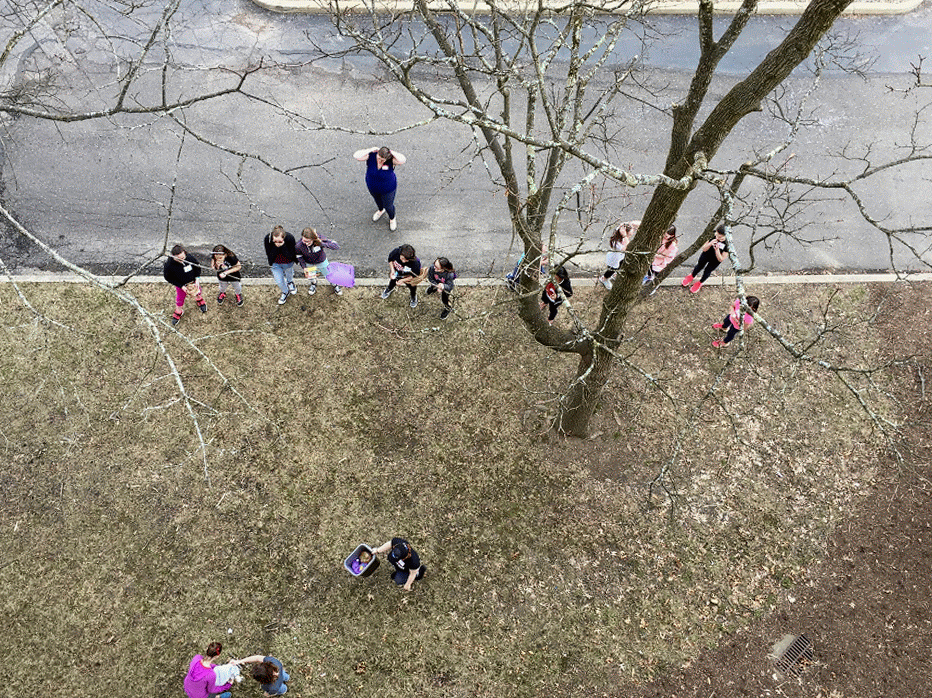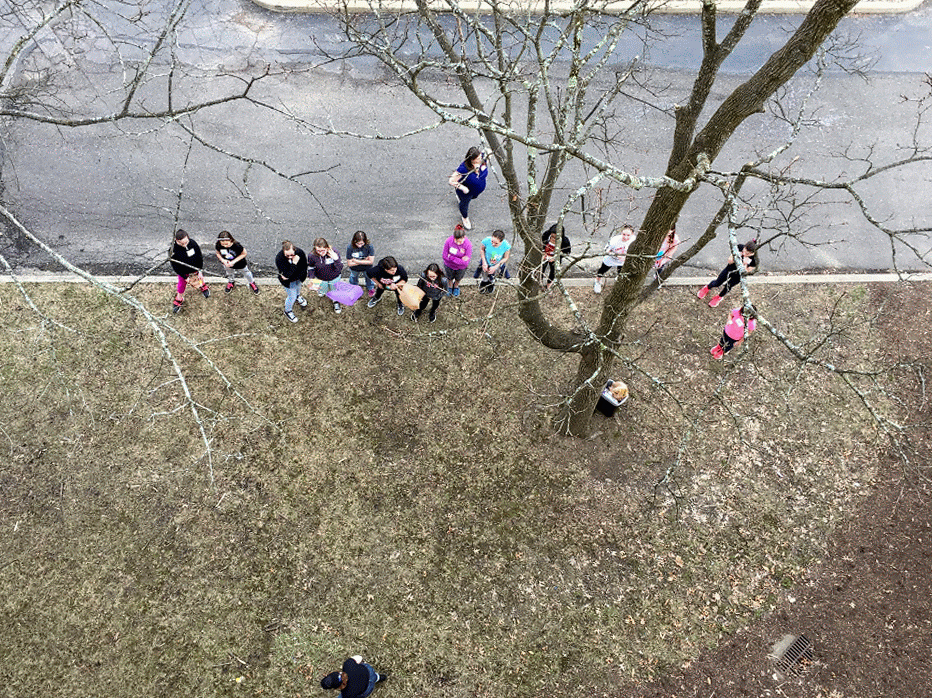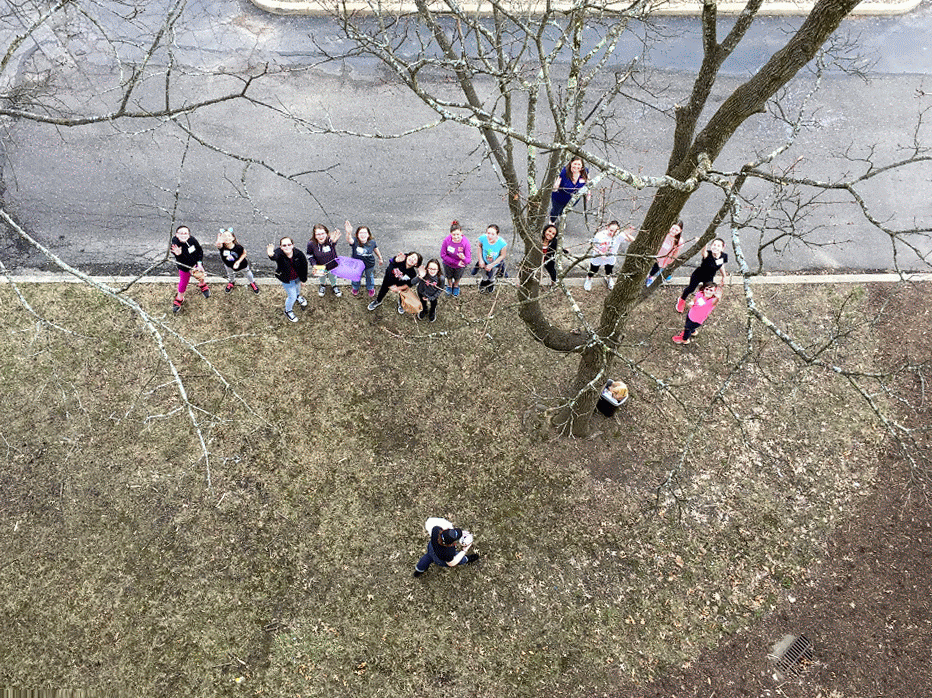
Most of the eggs this year made it! Lesson learned = parachutes really work!










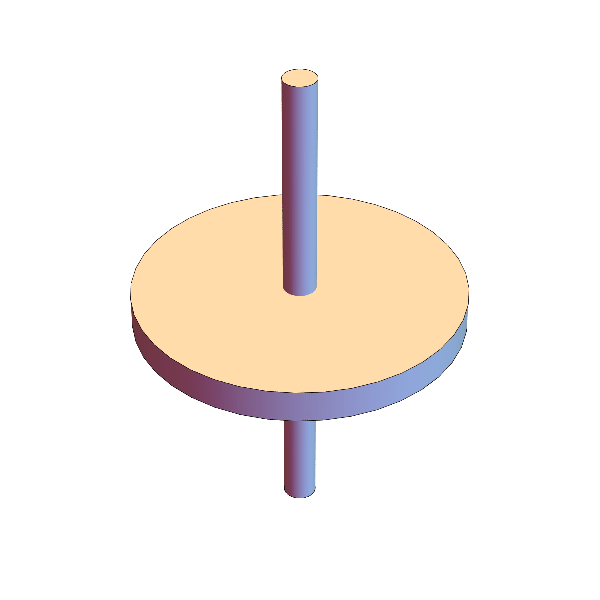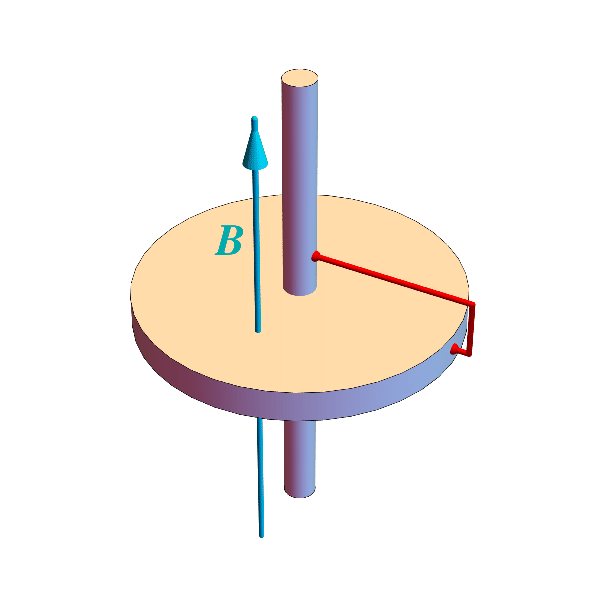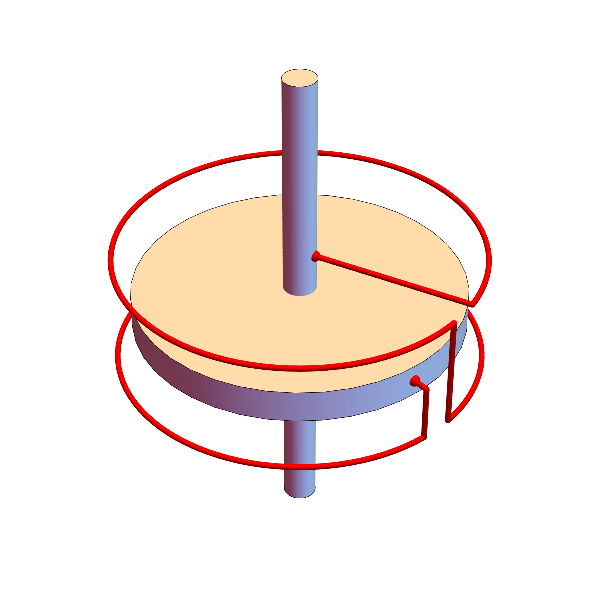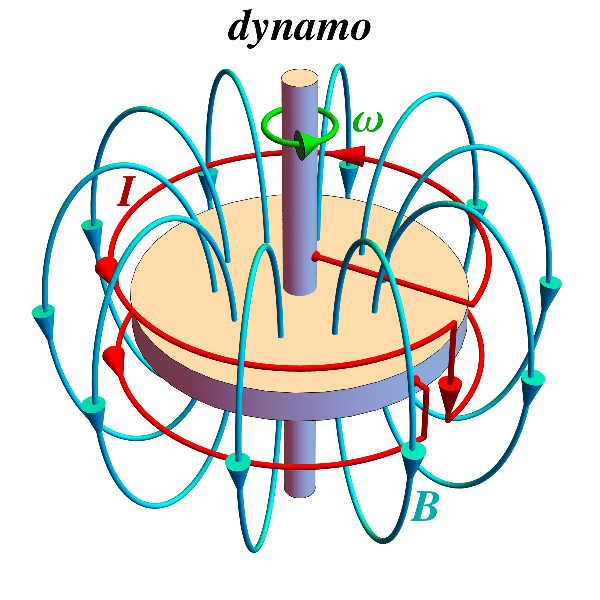




















Thanks, Mark! I enjoy reading your posts as well.