Our TV is broken, so Aunt Nora invites us to her apartment. (Aunt Nora isn’t really our aunt, but she introduced our parents to each other, so that’s what we call her.) My brother Jim and I lie on the floor close to the TV, while the adults sit on the couch. We watch NBC not CBS, so we miss Cronkite’s commentary. The late afternoon video is a simple animation; the famous 16-mm film — only later synchronized with the audio — would return to Earth with the astronauts 4 days later.
The tension is palpable. The cartoon lander reaches the surface at the expected time, but Aldrin’s monotone readouts continue. Absence of video heightens the audio. Mission control radios “60 seconds” of fuel remaining. Then “30 seconds”. I hold my breath. At last, Aldrin reports “Contact light” — we have touched the moon — followed by Armstrong’s famous, “Houston … Tranquility Base here, the Eagle has landed”. Of the landing site, my mother observes, “They’ve already named it”.
No one wants to cook, so we go to McDonald’s for dinner. As we drive, I see a small shop with photos of the three astronauts in its window. The streets are still. The world seems stopped.

10:56:15 PM EDT, Sunday, July 20, 1969.
As Collins orbits the moon solo, Armstrong and Aldrin forgo a scheduled sleep period, moving forward the moonwalk. Finally video — live from the surface of the moon — shows a LEM landing leg, first inverted but quickly rectified. Armstrong describes the surface as “almost like a powder”. Again I hold my breath, a lump in my throat. “Okay. I’m going to step off the LEM now … that’s one small step for [a] man, one giant leap for mankind.” I don’t hear the indefinite article, but I immediately grasp the meaning. Armstrong reads the plaque, “We came in peace for all mankind”. Aldrin practices locomotion, which “would get rather tiring”. The president phones. We leave Aunt Nora’s as the astronauts prepare to return to the LEM.
The next morning I sit on my living room floor reading two newspapers: The New York Times, with its simple banner headline “Men Walk on Moon” — which still brings me tears of joy, triumph, and wonder as I write this 50 years later — and the local newspaper, with its astonished “Now Do You Believe!”.

My Monday morning newspapers, July 21, 1969.
 Because I had time, I chose to walk/hike the 7 miles from the train station in the next larger town Goslar to the hotel. It was wonderful and my most relaxing travel to a conference site ever. Because of the rain in the last days, some parts where pretty wet and some streams got larger than usual. But other people ‘built’ already crossings.
Because I had time, I chose to walk/hike the 7 miles from the train station in the next larger town Goslar to the hotel. It was wonderful and my most relaxing travel to a conference site ever. Because of the rain in the last days, some parts where pretty wet and some streams got larger than usual. But other people ‘built’ already crossings. One thing I remembered, after it was too late: A shortcut is not always the best path to take. If you save on distance traveled in a mountainous regions you could pay with an increase in slope! Not surprising but interesting to realize.
One thing I remembered, after it was too late: A shortcut is not always the best path to take. If you save on distance traveled in a mountainous regions you could pay with an increase in slope! Not surprising but interesting to realize.







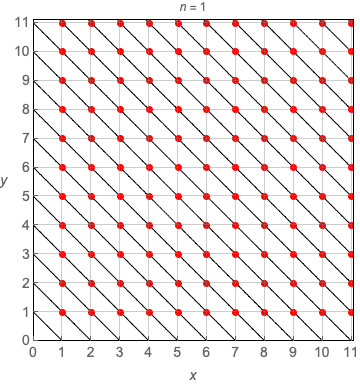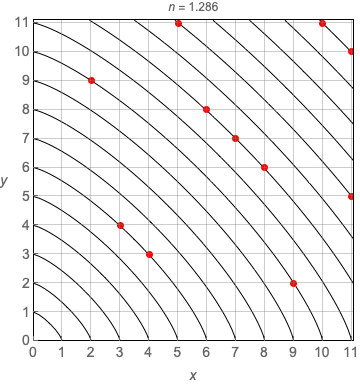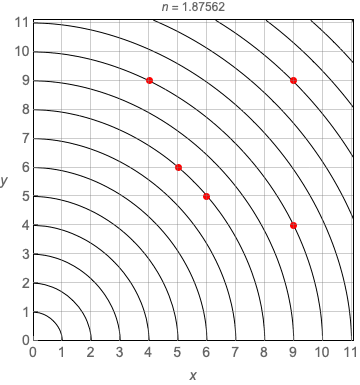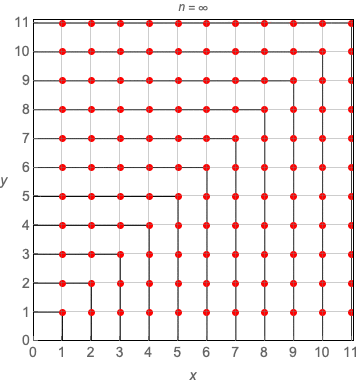




Thanks, Mark! I enjoy reading your posts as well.