This academic year has been thrilling: first nuclear fusion breakeven, now an ein stein!
Last week, a preprint at arxiv.org by David Smith et al. announced an “ein stein”, or one stone, a shape that forces a non periodic tiling of the plane, ending a half-century quest by many researchers, including me. A retiree and tiling enthusiast, Smith discovered the shape, which he calls “the hat”, and his professional colleagues provided mathematical and computational rigor.
In the 1960s, Robert Berger found a set of 20 426 tile-types that tile a plane but only non periodically — a wonderful mix of the expected and the surprising, a kind of visual music. By the 1970s, Roger Penrose has reduced that to just 2 tile-types, but a further reduction to just 1 tile-type proved elusive. Of Smith’s hat, the 91-year-old Roger Penrose reportedly observed, “It’s a really good shape, strikingly simple”, and I agree.
In the grayscale figure below, an underlying hexagonal grid emphasizes the hat’s construction. Given the prior theoretical and computational work that failed to find it, the hat is a surprisingly simple polykite formed from 8 kites or 4 double kites. In the color figure below, I color the hats according to their orientations (brightnesses) and reflections (hues), something I’ve dreamed of doing for many years, even in the absence of an ein stein.
You could order many flat paving stones shaped like the hat and translate, rotate, and flip them to non periodically tile your patio. I just might.
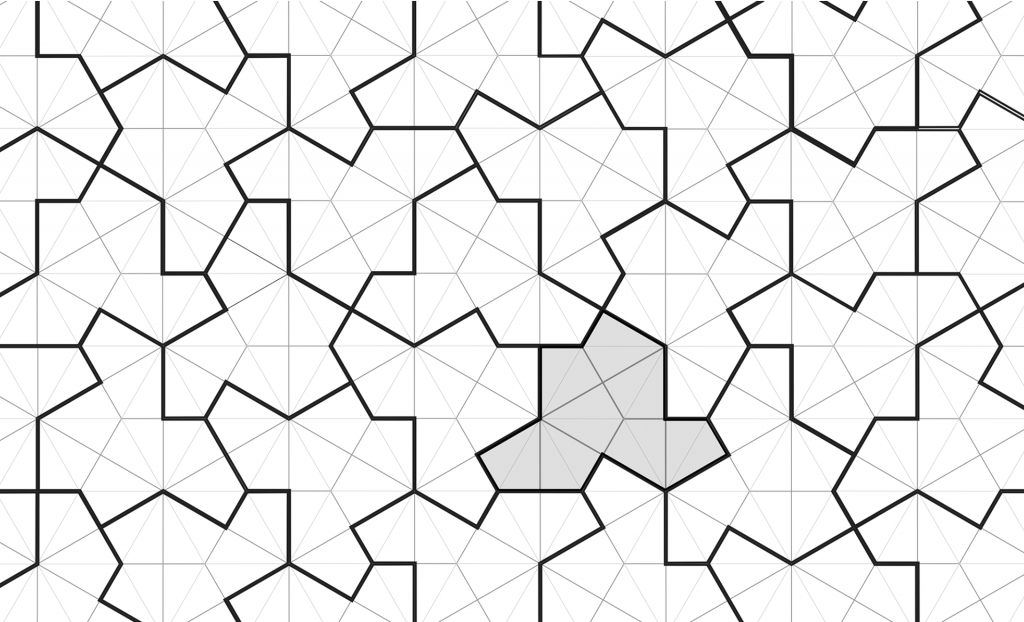
Figure 1.1 from David Smith et al. “An aperiodic monotile” emphasizing the underlying hexagonal symmetry of the hat, which is made from 8 kites or 4 double kites.
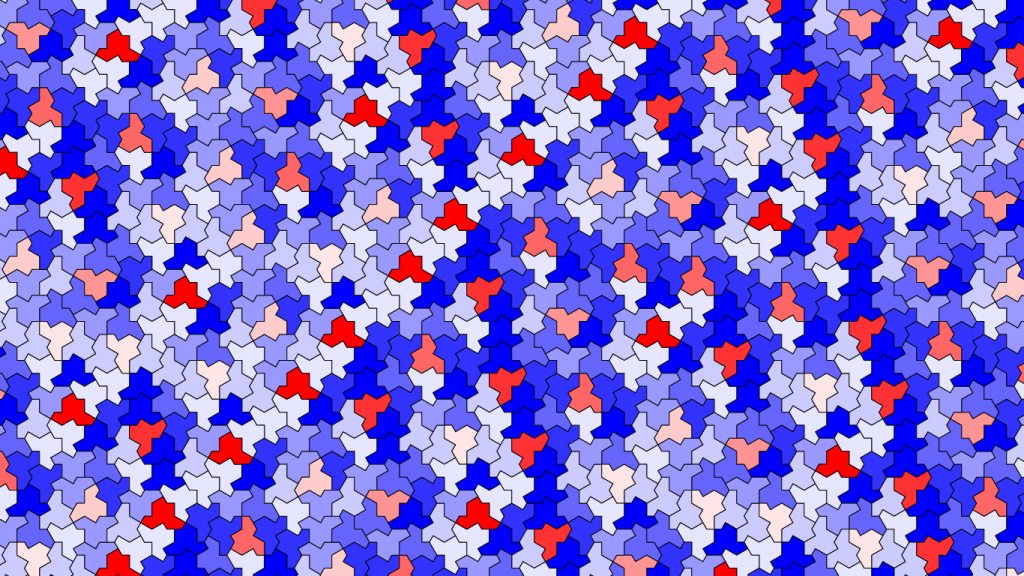
The hat forces a non periodic tiling of the plane, where brightnesses indicate 1 of 6 orientations and hues indicate 1 of 2 reflections. (Alternately, the orientations and reflections can all be considered rotations about axes perpendicular or parallel to the plane.)

Thanks, Mark! I enjoy reading your posts as well.